Someone was asking for a little hint on 2.28 from the second assignment, which asks for the smallest equilateral triangle with sides on a unit square.

First, convince yourself it is impossible for two of the vertices to be on the same side of the square.

Therefore, the three vertices are on three different sides. Hence two vertices will be opposite each other, with the third in-between, as in the diagram below

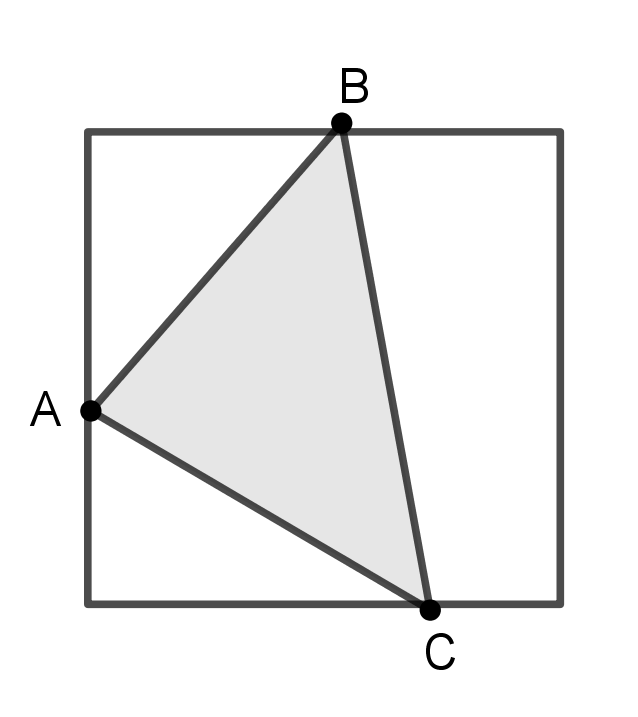
(Clearly this triangle is not drawn to scale.)

This should help visualize how to get an equilateral triangle with small area.