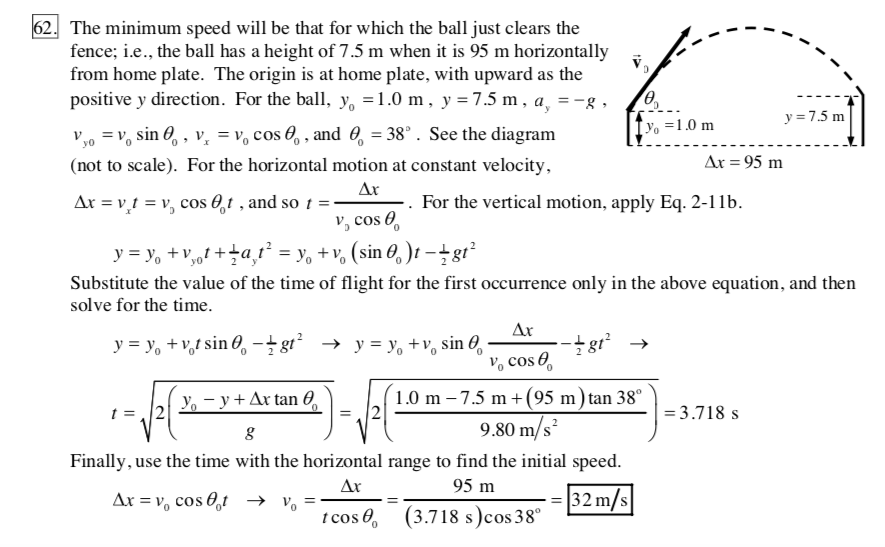
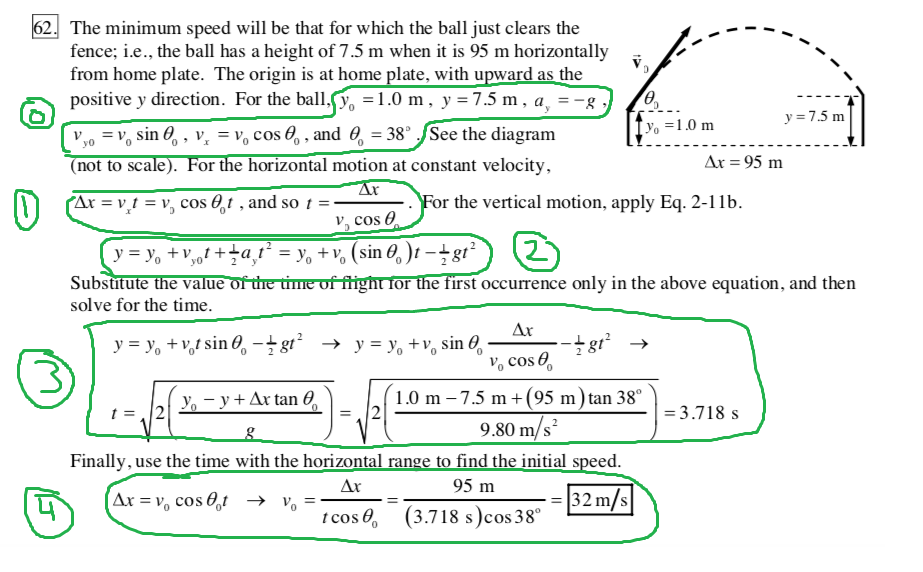
Let me use the solution you gave with parts labeled as reference.

First, let's walk through what I'd consider the "normal" solution. Proceed like the above up through and including equation (2) (ignore (3) and (4) for now).

Substituting the value for $t$ in (1) gives the equation: $$y = y_0 + v_0 \sin \theta_0 \cdot \frac{\Delta x}{v_0 \cos \theta_0} - \frac{1}{2}\cdot g\cdot \frac{(\Delta x)^2}{v_0^2\cdot \cos^2 \theta_0},$$ which simplifies to $$y = y_0 + \Delta x\cdot \tan \theta_0 - \frac{1}{2}\cdot g\cdot \frac{(\Delta x)^2}{v_0^2\cdot \cos^2 \theta_0}.$$ The only unknown here is $v_0$, so you can use this to solve for $v_0=32$ from here.

That being said, notice that in the middle term (which is the only substitution they made in (3) above) the v_0 terms cancel out, so actually that term is independent of the initial speed. So all that (3) and then (4) are doing is taking advantage of this fact to have slightly easier equations to work with, where you first solve for $t$ and for $v_0$ rather than doing it at once like we did above.

I'm a little bit confused by your other question. The issue with trying to deal with the height ($y$) directly is that we care about the height at a specific $x$ position. For example, even much smaller initial velocities will allow the ball to reach a height of $7.5$ meters, but it won't be at the correct $x$ position. Hope this helps, otherwise let us know if you have additional questions.