Online Course Discussion Forum
II-A Geometry
Hello!
For 1.19, how do you prove that the triangles on the ends of the trapezoid are 45-45-90? 1.28, I don't get the solution. For problem 2.6 b, Mr. David's internet was off, and I couldn't here the problem.For 2.14, I kept getting 60 degrees and 24 degrees. For 3.30, I don't get why the answer is y=3, I got y=1.5. FOr 4.26, can you give me the exact soluton? I did it the way the solution says on the quiz, but it did not give me the exact answers. Thank you!
Tina
In 1.19, remember that for trapezoids, we can often divide the trapezoid into a rectangle and two right triangles on the ends. Since both right triangles on the ends share the same height (the height of the trapezoid) as well as the same hypotenuse (of length $5\sqrt{2}$) those two triangles are congruent. From there you get their bases are length $5$ (since $5+10+5=20$) so they are right isosceles triangles. Hope that helps with the solution there.
For 1.28, it might help to look back at Problem 1.8, as that has the diagram with the equilateral triangle drawn. The key fact here is that in isosceles triangle ABC, if $\angle BAC = 20$, then the other two angles are both $80$ degrees. Hint: Note that $20+60 = 80$ as well.
2.6b is actually a special case of 2.26, so maybe it's better to look at that one. The diagram there is as shown below:
There are many different ways to solve from here (including using 2.6a). One alternate method: What types of shapes are formed if you extend lines $DP$, $EP$, and $FP$ to divide equilateral triangle $ABC$ into 6 pieces?
In 2.14, the 24 degrees (if the pentagon is outside the hexagon) is correct. Note this comes from an isosceles triangle with one angle measuring $360-120-108 = 132$ degrees. If the pentagon is inside the hexagon, the isosceles triangle should have one angle measuring $120-108 = 12$ degrees, right?
For 3.30, can you share a little of your work? Does the listed solution make sense or did you take a different approach?
For 4.26, consider the diagram below, which uses the information about the area of $GPFD$ and $EBHP$.
Since the full rectangle also has area $44$, we know that $(x+y)\times (12/x + 12/y) = 44$ as well. Since $y$ must be a factor of $10$, $y$ is 1, 2, 5, or 10. A little trial and error gives the answer from here. Hint: 44 can only be written as $2\times 22$ or $4\times 11$ as well, narrowing down the possibilities further.
Hope this helps! Let us know if you need any followup hints for ones like 3.30 or 4.26.
this is what i did:
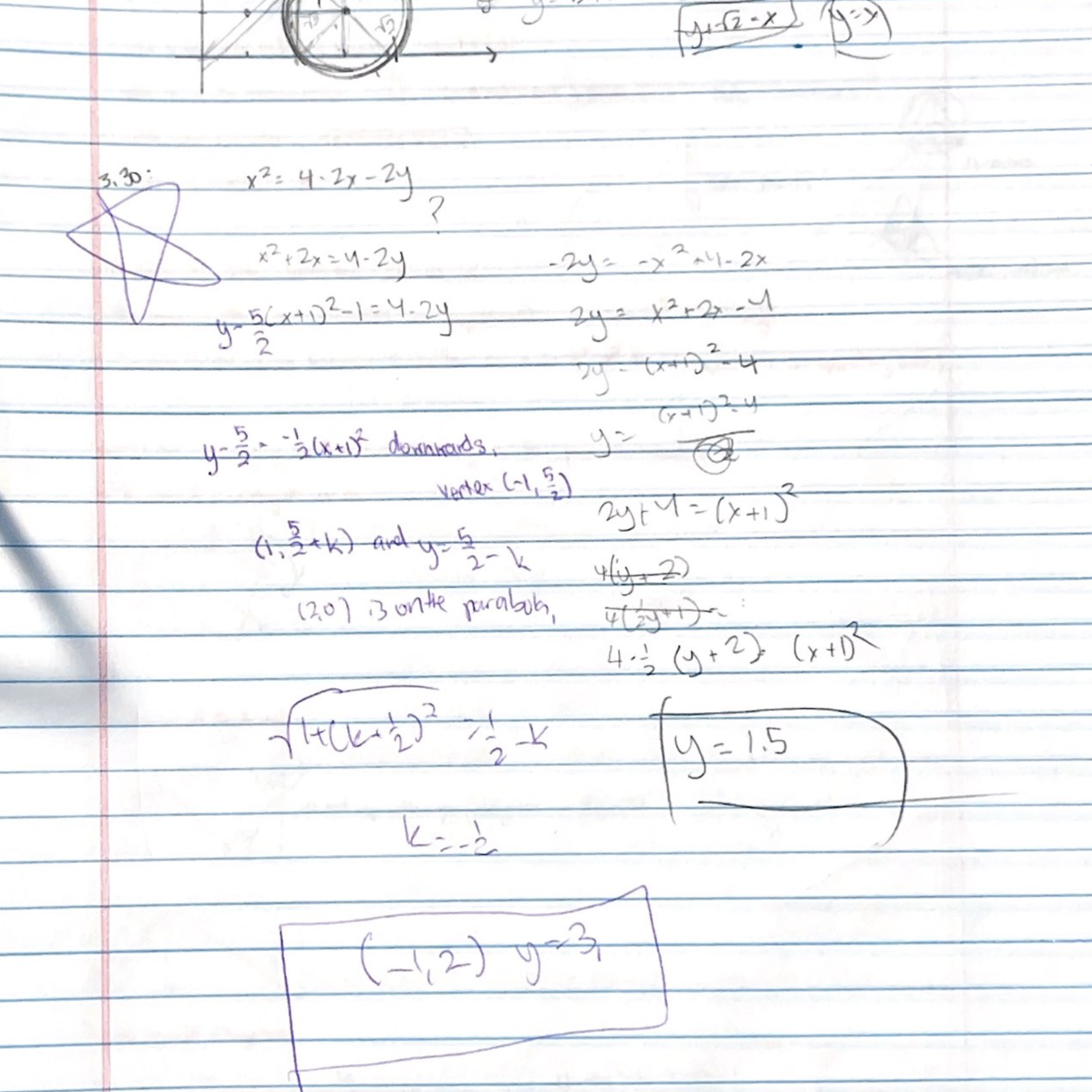Can you also give me the solution for problem 2.6b? I still don't really get it. Thank you so much for the responses!
For 3.30, you have the right idea to start. You can complete the square to write the parabola in vertex form. You have a little mistake there going from $2y = x^2+2x-4$ to $2y = (x+1)^2 - 4$, where it should instead be $2y = (x+1)^2 -5$ (double check by expanding this out). You're also off by a negative, so it actually should be $-2y = (x+1)^2 - 5$ which matches the $y = -\dfrac{1}{2}(x+1)^2 + \dfrac{5}{2}$. This means the vertex of the parabola is $(-1, 2.5)$.
Note, however, that the question is NOT asking for just the vertex. It is asking for the focus and directrix. Because the parabola opens downwards, the focus is (directly) below the vertex, and the directrix is above the vertex. More specifically, the focus (a point) must be of the form $(-1, 2.5+k)$ and the directrix (a line) must be of the form $y=2.5-k$ for some number $k$. Note we expect $k$ to be negative so that the focus is below the vertex.
The definition of a parabola is that all points on the parabola are the same distance from the focus as they are from the directrix. For ease, let's use the point $(0,2)$ from the parabola (although any point that isn't the vertex will work).
Distance from $(0,2)$ to the focus $(-1, 2.5+k)$:$$\sqrt{(0-1)^2 + (2-(2.5+k))^2}$$Distance from $(0,2)$ to the directrix $y=2.5-k$:$$|2-(2.5-k)|.$$Setting these equal will give $k = -0.5$ and therefore we know the focus and directrix.
For 2.6b and 2.26, consider extending the lines DP, EP, and FP as in the diagram below.
Note this divides the entire triangle ABC into three equilateral triangles (with light blue, pink, and orange side lengths) as well as three parallelograms. All the side lengths are color coded, note that each side equals 1 pink, 1 blue, and 1 orange, or $PD+PE+PF$ which is the key idea for the problem.
Hope these help!
Social networks