Online Course Discussion Forum
Geometry IIA 3.9 and 5.27
Hello!
For problem 3.9, Mr. John said that you have to have a tangent line to make it the shortest distance, why is it not directly to a point, like from C to A in the graph below? Why is it C to D?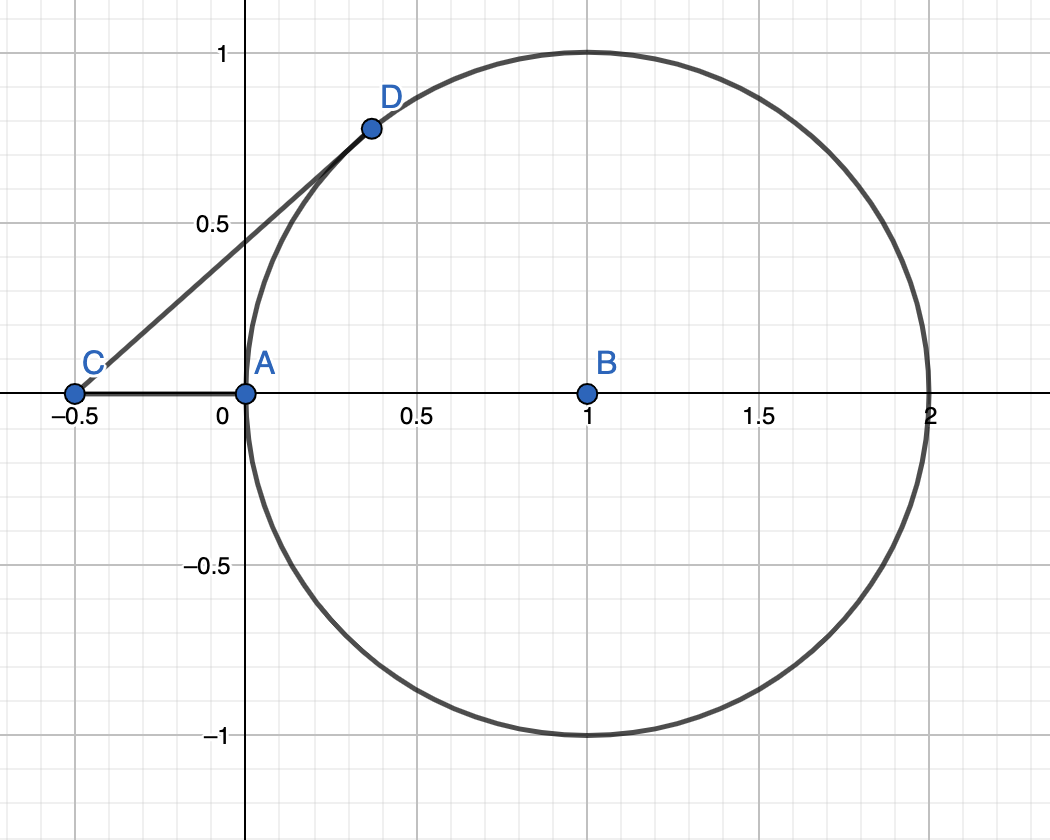
Also for problem 5.27, how do you get the lines for altitudes?
Thank you!
Tina Jin
For 3.9, the key here is that we want our entire path to start at the origin (point C in the diagram), fully travel around the circle, and come back to the origin.
IF our goal is just to get to the circle with a shortest distance, going from C to A is shorter. BUT if we're going around the circle, what do you do after A? Note the distance from C to A plus the distance from A to D (on the circle) is larger than the distance from C to D directly.
The idea then is that the tangent line is the "furthest" point on the circle that we can get to with a straight line. For example, if we tried to go to a point E further around the circle, the line CE would intersect the circle at another point, which doesn't work.
For 5.27, remember that an altitude is always perpendicular to the opposite base. Let's look at one example in more detail, the altitude through $A=(a,0)$ needs to be perpendicular to the line $BC$ (where $B=(0,b)$ and $C=(c,0)$). Note the slope of the line between points $B$ and $C$ is $\dfrac{-b}{c}$. Hence, we want a line with slope $\dfrac{c}{b}$ through the point $(a,0)$. This is$$y-0=\frac{c}{b}(x-a) \text{ or } y = \frac{c}{b}x - \frac{ac}{b}.$$Similar calculations give the other altitudes.
Hope this helps!
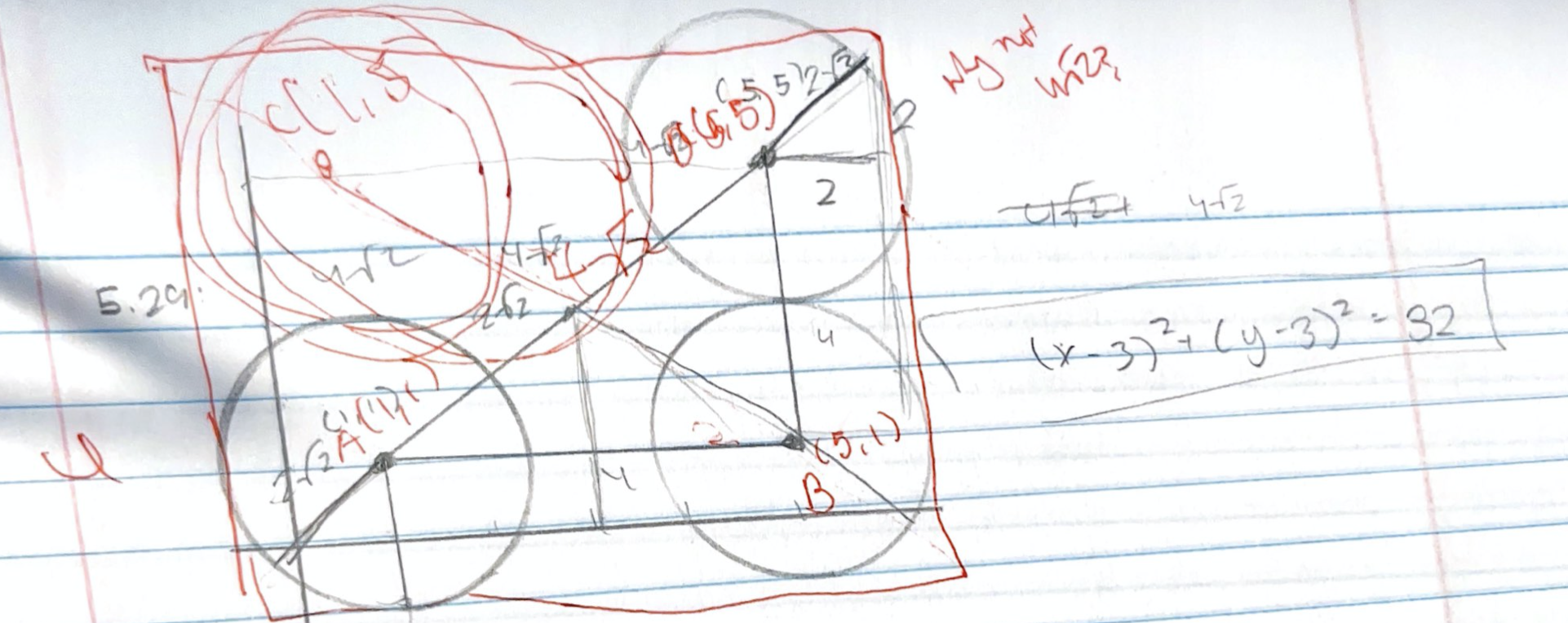
As in your picture, we can start by finding the distance of $AC$, which is$$\sqrt{(5-1)^2 + (5-1)^2} = \sqrt{32} = 4\sqrt{2}.$$Be careful however, because each of the 4 circles has a radius of $2$. So when we're finding the diameter of the big circle, it's equal to the distance from the bottom left edge of circle $A$ to the center (which is length $2$), plus the length of $AC$ (which is $4\sqrt{2}$), plus the distance from the center of circle $C$ to it's top right edge (also length $2$). This gives a diameter of $4+4\sqrt{2}$.
It looks like you may have mixed up the outside radiuses of the circles a bit. Hope this helps!
社交网络