Online Course Discussion Forum
MC III Number Theory 1.17,1.18,1.19
May I have some ideas on how to start these? They were not explained in class. Thank you!
For 1.18, consider x^2 = (-x)^2.
For 1.19,write the left and side as n(n + 1)/2.
Good luck.
I couldn't follow the solutions on AoPS for 1.17, they were too confusing
I tried $m=2,4$ and I figured all sums I tested don't work
but I can't figure out how to prove it for all m
maybe induction??
$\text{For 1.18}$,
$\text{I tried small values and they all showed that they were not complete residue systems.}$
I tried making $x^2$=$(-x)^2$ like you suggested but I don't really know what to do after that,
I'm figuring out what to do after making the left $\frac{n(n+1)}{2}$ for 1.19
Hi Alan,
To start, it might be good to revisit 1.16 and the extra problem we called 16.5 in the live class. The relevant portions start around 1 Hour and 18 Minutes in the Week 2 Recording. Note: the key idea here is pairing a number a with (m-a). Note (m-a) can also be through of as -a if we're working mod m.
For 1.17, the extra problem 16.5 is actually one easy way to prove the result.
For 1.18, the pairing trick mentioned above is also the key.
For 1.19, it's probably easiest to think of two cases, once for n even and one for n odd. Both cases are similar but it can be easier to think of them separately. As a further hint, if n is even, try to prove (n/2) divides (1^k+...+n^k) and (n+1) divides (1^k+...+n^k). Again pairing will be helpful.
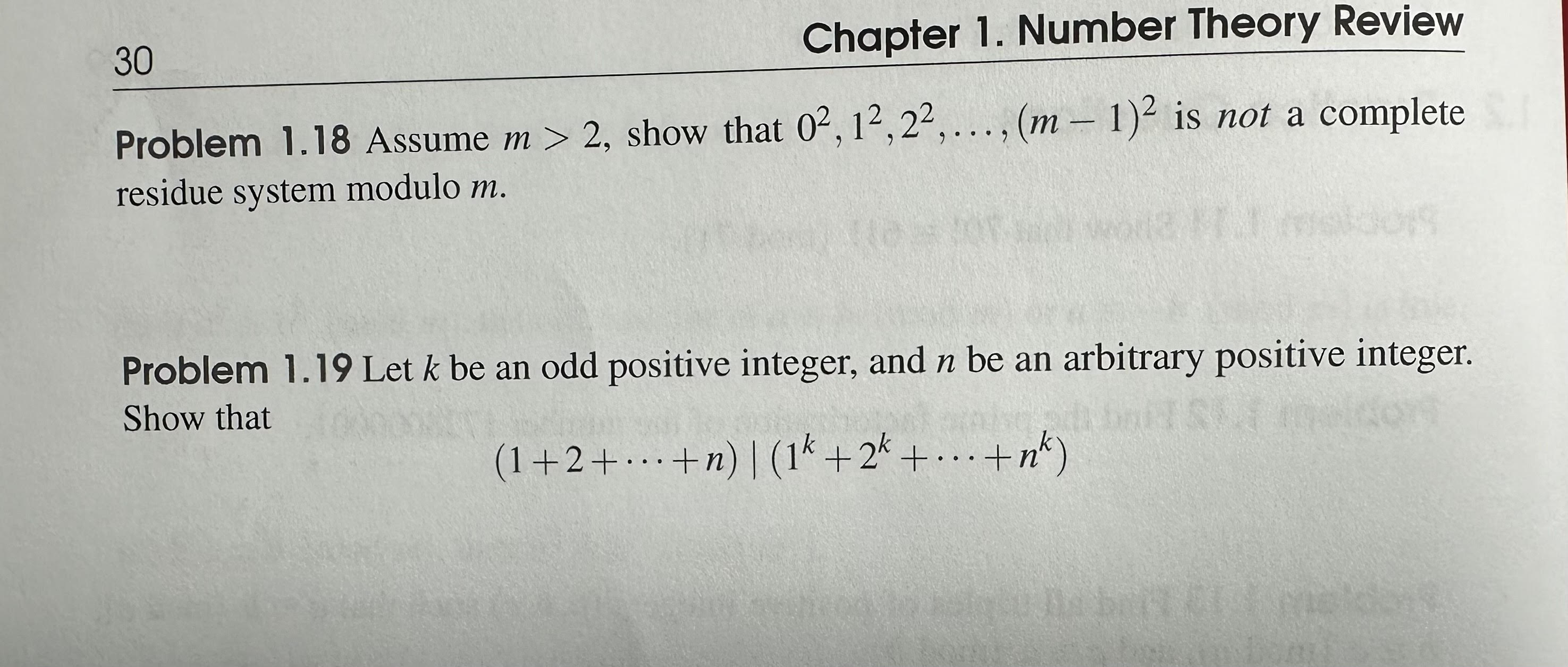

社交网络