Online Course Discussion Forum
Question about II-A Geometry 2020 2.29
Hello, I am reviewing my missed questions, and I don't get the explanation for this problem.
I am not sure how you can rotate triangle PDC around angle C 90 degrees and find angle PCQ is exactly half of that 90 degrees.
Can someone help me out?
Here is the question: Let ABCDABCD be a unit square and let P,QP,Q be on sides AD¯¯¯¯¯¯¯¯,AB¯¯¯¯¯¯¯¯AD¯,AB¯ respectively such that △APQ△APQ has perimeter 22. Find the measure of ∠PCQ∠PCQ.
In the diagram below we can see the rotated triangle $\triangle BCP'$.
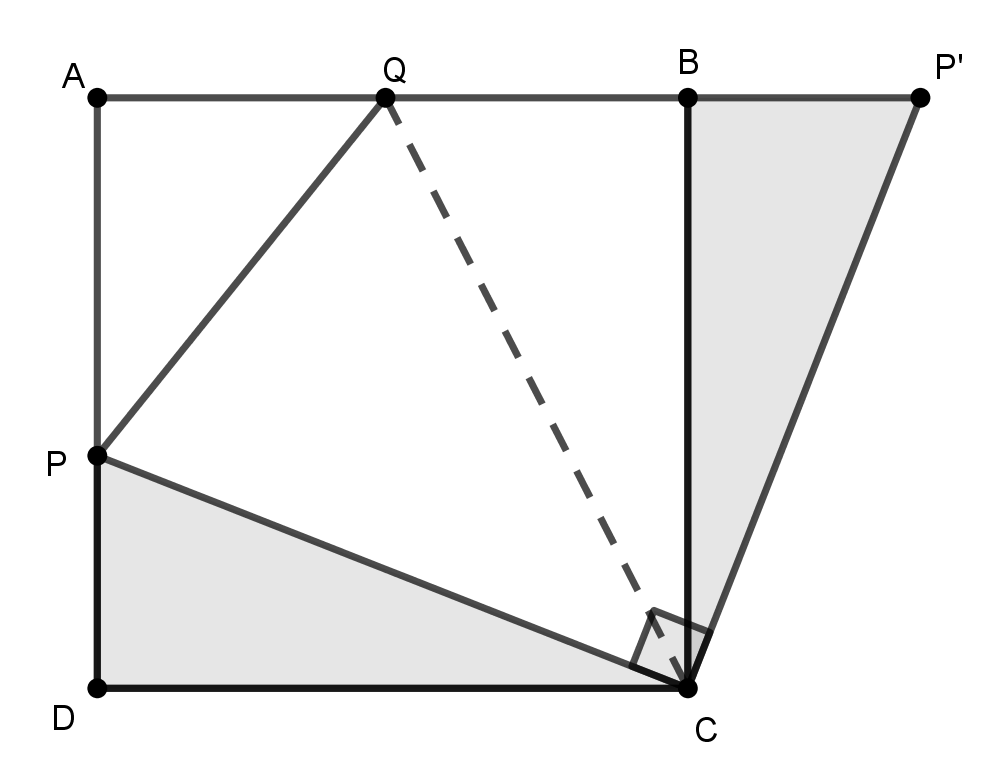
The key is noticing that since $ABCD$ is a unit square, $\triangle APQ$ has perimeter $2$, and $\triangle PDC \cong \triangle P'BC$, $\triangle PQC \cong \triangle P'QC$:
- $PC = P'C$
- $QC = QC$
- $QP = 2 - AQ - AP = (1-AQ) + (1- AP) = QB + DP = QB + BP' = QP'$
In particular, $\angle P'CQ = \angle PCQ$. Since we rotated $90^\circ$, $\angle PCP' = 90^\circ$, and $\angle PCP' = \angle PCQ + \angle P'CQ$, so $\angle PCQ = 90^\circ \div 2 = 45^\circ$.
社交网络