Online Course Discussion Forum
math challenge II B
In the third video lecture, problem 3.9. The teacher wrote: note: x*ybar +y*xbar = 2*Real(xy)
But I calculated and it's not equal.
Also, does my method to approach the question correct?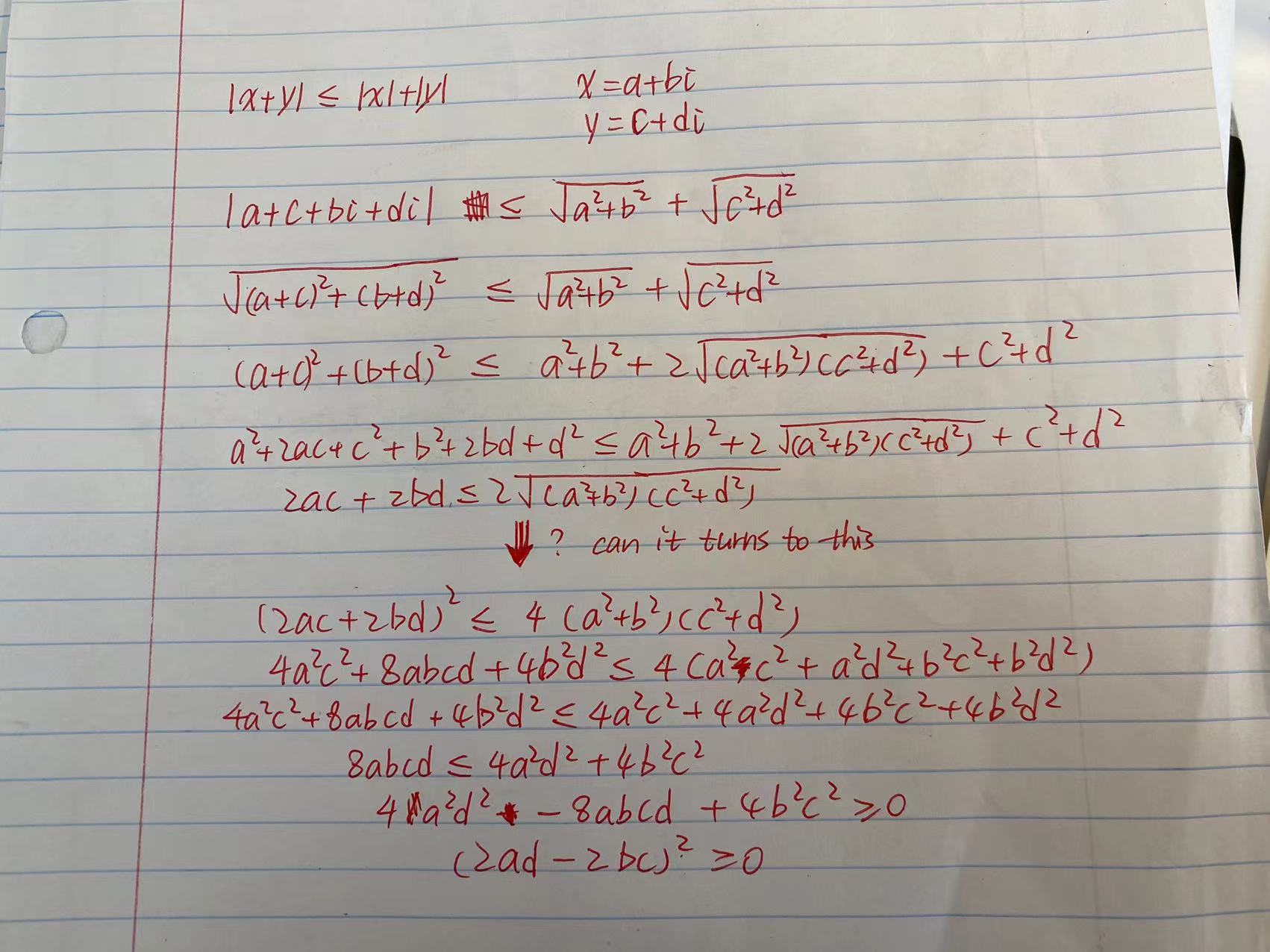
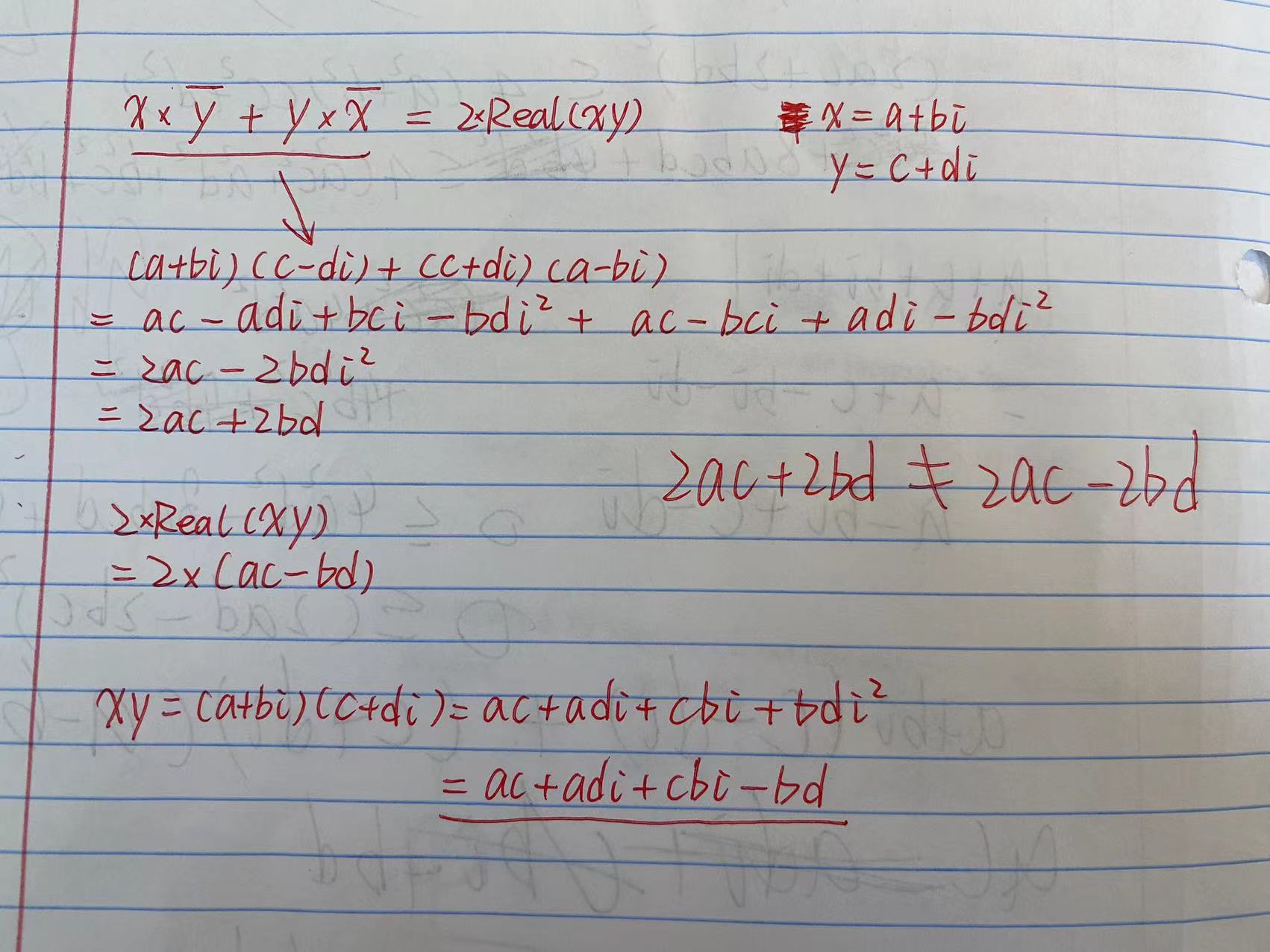
(For context: this question asks to prove the triangle inequality $|x+y| \leq |x| + |y|$ where $x$ and $y$ are complex numbers.)
Hi Zitian,
You are right that the statement that $$x\overline{y}+y\overline{x}=2\text{Re}(xy)$$ is incorrect. The right hand side should be $2\text{Re}(x\overline{y})$. The rest of the proof is not affected by this change. Sorry for the confusion. The teachers are planning to fix this error soon.
Your approach is excellent! Very nice algebraic transformations.
To be mathematically rigorous, you can rewrite the whole solution in reverse order (start from the last line, and write backwards until you get $|x+y|\leq |x|+|y|$). Or keep your solution the way it is, and just add one statement at the end: "Since every step is reversible, we have proved the triangle inequality."
Social networks